Category:Electron-phonon interactions

In many systems it suffices to treat the electronic and vibrational degrees of freedom (phonons) separately, because electrons are much faster than the motion of nuclei. This treatment is approximate and can be corrected by including electron-phonon coupling. This entails the coupling of the two systems, while still treating the two systems separately. In fact, phonon scattering is the prevalent effect in a wide range of applications such as mobility of semiconductors or conductivity of metals at room temperature.

The inclusion of the effects of the ionic degrees of freedom in the electronic structure is important in the determination of many physical observables such as the bandgap, spectral functions, electronic conductivity, Seebeck coefficient or electronic thermal conductivity to name a few.

One can use different approaches to include electron-phonon coupling:

• statistically averaging observables during a molecular dynamics calculation,
• stochastically sampling the ionic degrees of freedom, or
• using many-body perturbation theory (available as of VASP 6.5.0).

In this page we outline the two later approaches, their advantages and disadvantages and range of application. For a comprehensive overview of the theory of electron-phonon coupling, we recommend the review by F. Giustino ^[1].

Stochastic displacements approach

The stochastic approach allows obtaining the bandgap renormalization and an approximation of the electronic spectral function due to the ionic degrees of freedom under the static approximation using a supercell approach. This has the advantage that it can be easily implemented and used with different levels of theory to describe the electronic states, such as different exchange-correlation functionals or even the GW approximation.

This approach requires the knowledge of the phonons on a supercell. These are then used to displace the atoms along a set of random or a single specially chosen direction ^[2] with the displacement length determined by the temperature of the ionic system. The bandgap can be directly obtained by averaging over the set of randomly displaced supercells, or from the aforementioned special displacement pattern.

• Electron-phonon interactions from Monte-Carlo sampling

Many-body perturbation theory

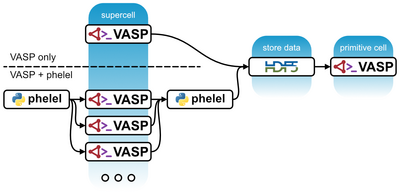
Another approach to include electron-phonon coupling employs the methods and language of many-body perturbation theory, where the coupling is included as a perturbation of the electronic or phononic states. In the case of the perturbation of the electronic states, we can access the bandgap renormalization as well as electronic transport coefficients with the inclusion of phonon scattering.

This approach entails computing the electron-phonon matrix element and the phonon-induced electron self-energy. Within the framework of density-functional theory this requires the knowledge of the change of Kohn-Sham potential with an ionic perturbation as well as the initial and final electronic Kohn-Sham states. The calculation is done in two steps:

Step 1: Electron-phonon potential from supercells

In the first step, we obtain the derivatives of the Kohn-sham potential with respect to the ionic displacements

${\displaystyle \partial_{I \alpha} V (\mathbf{r}) = \frac{\partial V(r)}{\partial R_{I\alpha}} }$

with ${\displaystyle I}$ the ion index and ${\displaystyle \alpha}$ denoting the Cartesian direction in which it is displaced. The main output file is phelel_params.hdf5, which is required for computing the matrix elements in the next step.

• How to compute the electron-phonon potential from supercells

Step 2: Physical observables (or electron-phonon matrix elements)

To compute the physical observables, the phonon-induced electron self-energy is computed in the primitive cell. The main tag that provides convenient defaults depending on the observable of interest is ELPH_MODE. :The computation of the self-energy requires evaluating the electron-phonon matrix elements

${\displaystyle g_{mn \mathbf{k}, \nu \mathbf{q}} \equiv \langle \psi_{m \mathbf{k} - \mathbf{q}} | \partial_{\nu \mathbf{q}} V | \psi_{n \mathbf{k}} \rangle. }$

By default, we avoid writing the matrix elements, because it is a huge data set which is distributed for optimal use of the computational resources.

For details on the setup and practical advice, we recommend reading

• Bandgap renormalization due to electron-phonon coupling
• Transport coefficients including electron-phonon scattering
• See the ELPH_DRIVER tag to obtain the electron-phonon matrix elements for further post-processing.

References