|
|
| Line 50: |
Line 50: |
| </math> | | </math> |
|
| |
|
| %\[
| | the Hamiltonian changes only minimally |
| %\left( \begin{array}{c} \mid \Psi^{\uparrow} \rangle \\ \mid \Psi^{\downarrow} \rangle \end{array} \right)
| |
| %\rightarrow
| |
| %\left( \begin{array}{c} e^{-i\bf q \cdot \bf r / 2} \mid \Psi^{\uparrow} \rangle \\ e^{+i\bf q \cdot \bf r / 2}\mid \Psi^{\downarrow} \rangle \end{array} \right)
| |
| %\]
| |
|
| |
|
| the Hamiltonian changes only minimally
| | :<math> |
| \[
| |
| \left( \begin{array}{cc} | | \left( \begin{array}{cc} |
| H^{\alpha\alpha} & V^{\alpha\beta}_{\rm xc} \\ | | H^{\uparrow\uparrow} & V^{\uparrow\downarrow}_{\rm xc} \\ |
| V^{\beta\alpha}_{\rm xc} & H^{\beta\beta} \end{array}\right) | | V^{\downarrow\uparrow}_{\rm xc} & H^{\downarrow\downarrow} \end{array}\right) |
| \rightarrow | | \rightarrow |
| \left( \begin{array}{cc} | | \left( \begin{array}{cc} |
| H^{\alpha\alpha} & V^{\alpha\beta}_{\rm xc} e^{-i\bf q \cdot \bf r} \\ | | H^{\uparrow\uparrow} & V^{\uparrow\downarrow}_{\rm xc} e^{-i\bf q \cdot \bf r} \\ |
| V^{\beta\alpha}_{\rm xc}e^{+i\bf q \cdot \bf r} & H^{\beta\beta} \end{array}\right) | | V^{\downarrow\uparrow}_{\rm xc}e^{+i\bf q \cdot \bf r} & H^{\downarrow\downarrow} \end{array}\right) |
| \]
| | </math> |
|
| |
|
| where in $H^{\alpha\alpha}$ and $H^{\beta\beta}$ the kinetic energy of a plane wave component changes to | | where in $H^{\uparrow\uparrow}$ and $H^{\downarrow\downarrow}$ the kinetic energy of a plane wave component changes to |
|
| |
|
| :<math> | | :<math> |
Revision as of 13:13, 6 July 2018
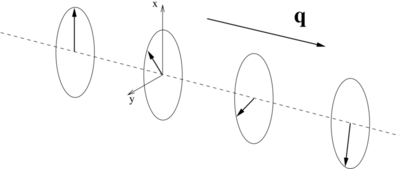
Generalized Bloch condition
Spin spirals may be conveniently modeled using a generalization of the Bloch condition (set LNONCOLLINEAR=.TRUE. and LSPIRAL=.TRUE.):
![{\displaystyle
\left[ \begin{array}{c} \Psi^{\uparrow}_{\bf k}(\bf r) \\
\Psi^{\downarrow}_{\bf k}(\bf r) \end{array} \right] = \left(
\begin{array}{cc}
e^{-i\bf q \cdot \bf R / 2} & 0\\
0 & e^{+i\bf q \cdot \bf R / 2} \end{array}\right) \left[
\begin{array}{c} \Psi^{\uparrow}_{\bf k}(\bf r-R) \\
\Psi^{\downarrow}_{\bf k}(\bf r-R) \end{array} \right],
}](/wiki/index.php?title=Special:MathShowImage&hash=d87ea979867db8fcc85d3f002be8e039&mode=mathml)
i.e., from one unit cell to the next the up- and down-spinors pick up an additional phase factor of  and
and  , respectively,
where R is a lattice vector of the crystalline lattice, and q is the so-called spin-spiral propagation vector.
, respectively,
where R is a lattice vector of the crystalline lattice, and q is the so-called spin-spiral propagation vector.
The spin-spiral propagation vector is commonly chosen to lie within the first Brillouin zone of the reciprocal space lattice, and has to be specified by means of the QSPIRAL-tag.
The generalized Bloch condition above gives rise to the following behavior of the magnetization density:

This is schematically depicted in the figure at the top of this page:
the components of the magnization in the xy-plane rotate about the spin-spiral propagation vector q.
Basis set considerations
redefining the Bloch functions


the Hamiltonian changes only minimally

where in $H^{\uparrow\uparrow}$ and $H^{\downarrow\downarrow}$ the kinetic energy of a plane wave component changes to



